THE FERMAT POINT

By Dario Gonzalez Martinez

This point, also called the Torricelli point, is the solution of the problem:

“Given a triangle ABC, find a point P such that the sum of distances from P to each triangle’s vertex is a minimum, that is, the sum |PA| + |PB| + |PC| is as small as possible.”

Although this is the most popular version of the problem, some mathematicians say that the original problem with which Fermat challenged Torricelli (that is why the point is also known as Torricelli point) was:

“Given three points in a plane, find a fourth point such that the sum of its distances to the three given points is a minimum”

Although there are several ways to solve this problem, I would like to refer one of the most known since it remembered me a problem that my calculus instructor presented to me in a test. This problem is a typical optimization problem in calculus which is as follow:

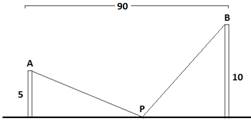
“There are two posts separated for 90 meter each other. Each post is 5 and 10 meter tall, respectively. It is necessary to put a support between the two posts that holds the two posts by cables that extend from the support to each post’s top. How far from each post the support must be put to spend a minimum amount of cable.”

Here is figure of the problem:

Figure 1

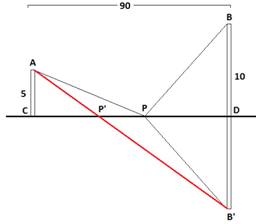
The instructor wanted us to use calculus tools to solve the problem. However, one really smart classmate came up with the following idea: he reflected the tallest post around the ground and drew the line AB’ shown in figure 2 below.

Figure 2

We all know that the shortest distance between two points on a plane is a straight line. Thus, it just remain for him to find the ratio between triangles ACP’ and DB’P’ (since they are similar) to find the location of the support. That was a great idea since you can avoid a tricky calculus way. I just presented this story since there is a similar idea involved in the solution that I would like to show for Fermat point.

SOLUTION FOR FERMAT-TORRICELLI CHALLENGE

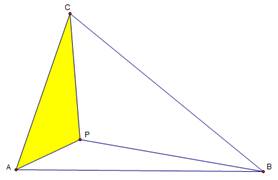
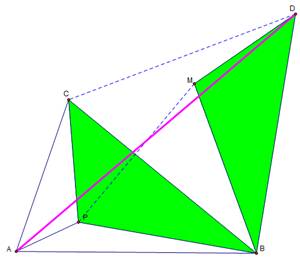
Let me remember the problem. Given a triangle ABC, we need to find a point P such that the sum of distances from P to each triangle’s vertex is a minimum. To this end, consider a triangle ABC which will be any triangle with each angle less than 120 degrees (I will refer to the case when the triangle has a 120-degree angle or more later). Let P be a point connected with each vertex of the triangle ABC as shown in figure below:

Figure 3

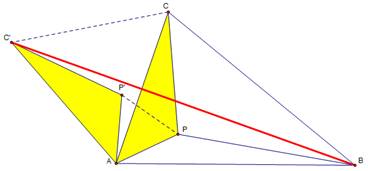
Now we rotate triangle APC 60 degree around A, and we obtain triangle AP’C’ as follow in figure 4 below:

Figure 4

We must note some facts here. First triangles ACC’ and APP’ are equilateral since

Triangle ACC’: Angle C’AC is 60 degrees by construction. We know that AC = AC’ then angles AC’C and ACC’ must be equal. Since angle C’AC is 60 degrees then angles AC’C and ACC’ are also 60 degrees.

Triangle APP’: Angle P’AP is 60 degrees by construction. We know that AP = AP’ then angles AP’P and APP’ must be equal. Since angle P’AP is 60 degrees then angles AP’P and APP’ are also 60 degrees.

Second, since triangle APP’ is equilateral, then PA = PP’. Thus, PA + PB + PC = PP’ + PB + P’C’.

Third, since the broken line BPP’C’ cannot be shorter than the straight line BC’.

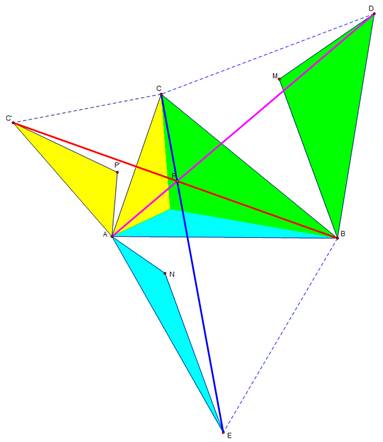
Therefore, PA + PB + PC = PP’ + PB + P’C’ will be a minimum if and only if P lies on BC’. Of course this reasoning is not enough to find the exactly location of P yet. However, we can make a similar reasoning, but now we rotate triangle CPB 60 degree (clockwise) around B. so, we now consider the figure 5 below:

Figure 5

We can note here similar facts that above. First, triangles BCD and BPM are equilateral since

Triangle BCD: Angle CBD is 60 degrees by construction. We know that BC = BD then angles BDC and BCD must be equal. Since angle CBD is 60 degrees then angles BDC and BCD are also 60 degrees.

Triangle BPM: Angle PBM is 60 degrees by construction. We know that BP = BM then angles MPB and PMB must be equal. Since angle PBM is 60 degrees then angles MPB and PMB are also 60 degrees.

Second, since triangle BPM is equilateral, then PB = PM. Thus, PA + PB + PC = PA + PM + MD.

Third, since the broken line APMD cannot be shorter than the straight line AD.

Therefore, PA + PB + PC = PA + PM + MD will be a minimum if and only if P lies on AD.

We could make a similar reasoning for triangle ABP, and we will finally find that P should be lie on CE to make PA + PB + PC be a minimum. Thus, P must lie on the intersection of BC’, AD and CE since P lies on BC’, AD and CE at the same time. This is shown in figure 6 below:

Figure 6

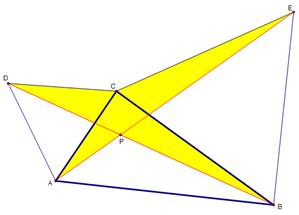
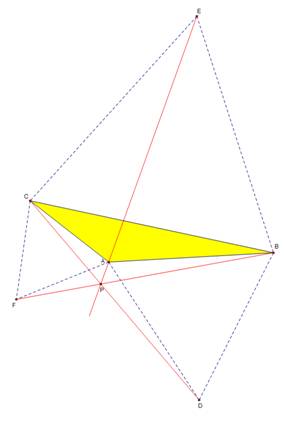
We could call BC’, AD and CE diagonals of polygon AEBDCC’. It would be interesting to know the angle between two of these diagonals. Consider a triangle ABC as we agreed (a triangle with each interior angle less than 120 degrees), and let AE and BD be diagonals and let P be the Fermat point as follow:

Figure 7

It is easy to note that triangles BDC and AEC are congruent by SAS criterion. Let angle ACB be q degrees, and you know that angles ACD and BCE are 60 degrees since triangles ACD and BCE are equilateral. Therefore, angle DCB and ACE are both equal to 60 + q degrees. Also, AC = DC and CB = CE since triangles ACD and BCE are equilateral, respectively. Thus, by SAS criterion triangles BDC and AEC are congruent.

So, triangle AEC is just a 60 degrees rotation (anti-clockwise) of triangle BDC. Then, angle APD must be 60 degrees, so angle APB is 120 degrees. This result gives us two important considerations about Fermat point. First, we can conclude an easy rule to construct the Fermat point. Second, we can discuss the case when triangle ABC has an angle equal to or more than 120 degrees.

Fermat point construction

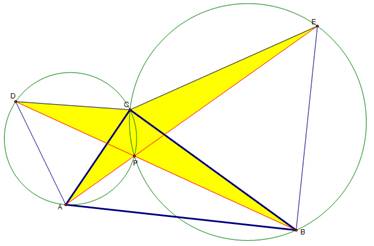
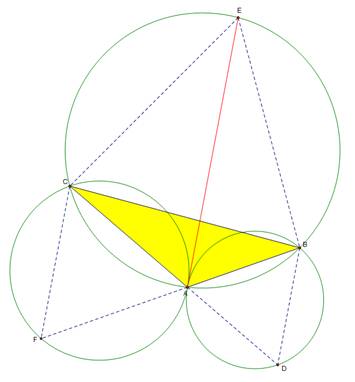
We will consider again the triangle ABC in figure 7, but this time, by using the central angle theorem, we will construct the circle that inscribes the angles APD and ACD, and the circle that inscribes the angles BPE and BCE. The result is show in figure 8 below:

Figure 8

Thus, the Fermat point construction is as follow:

Given a triangle ABC that has no angle equal to or more than 120 degrees. Then,

1) Construct an external equilateral ACD triangle on the side AC of the given triangle.

2) Construct the diagonal DB.

3) Construct the circumcircle of triangle ACD.

4) The intersection point F between the circumcircle and the diagonal DB is the Fermat point.

Figure 9

Fermat point when DABC has an angle equal to or more than 120 degrees

Consider again figure 7. We concluded that angle APB is 120 degrees, and it holds for any triangle because of the construction of point P.

Now consider a triangle ABC such that the angle at A is 120 degrees. The figure 10 below shows the triangle ABC, the equilateral triangles on its sides, the circumcircle for each equilateral triangle, and the diagonal AE.

Figure 10

According to figure 10 and the construction of Fermat point that we already explained, when DABC has a 120-degree angle, then Fermat point lies on the vertex of such angle. This makes sense since, as we already saw, two diagonals make a 120-degree angle, and by central angle theorem we observe that angles DAE, FAE, and CAB are equal to 120 degrees.

It just remains to analyze what occurs with Fermat point when DABC has an angle larger than 120 degrees. To this end, we will consider figure 11 below where we see DABC which has an angle larger than 120 degrees at A. Also, we can see the equilateral triangles on each side of DABC and the diagonals AE, BF, and CD.

Figure 11

Once again, the angle CPB is 120 degrees, but this time P is not the Fermat point. The reason is simple,

Thus, the Fermat point remains at A when the angle at A is larger than 120 degrees. In summary, the solution for the problem:

“Given a triangle ABC, we need to find a point P such that the sum of distances from P to each triangle’s vertex is a minimum”

CASE 1	SOLUTION
DABC has NO angle equal to or larger than 120 degrees	1) Construct an external equilateral ACD triangle on the side AC of the given triangle. 2) Construct the diagonal DB. 3) Construct the circumcircle of triangle ACD. 4) The intersection point F between the circumcircle and the diagonal DB is the Fermat point.
DABC has an angle equal to or larger than 120 degrees	The Fermat point lies at the vertex with the angle of 120 degrees or larger.